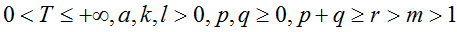Global Existence and Blow-up Properties of Solutions for a Degenerate Parabolic Equation with Nonlinear Memory and Absorptions
[1]
Zhoujin Cui, Jiangsu Maritime Institute, Nanjing, China.
In this paper by using upper and lower solution theorem, the existence of a unique classical nonnegative solution is established and the sufficient conditions for the solution that exists globally or blows up in finite time are obtained for the degenerate parabolic problem
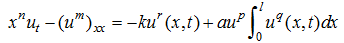
in
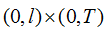
where
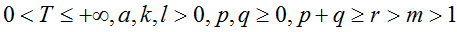
Blow-up, Nonlocal Source, Degenerate Parabolic Equation
[1]
C. V. Pao, Blowing-up of solution for a nonlocal reaction-diffusion problem in combustion theory, J. Math. Anal. Appl. 162 (2) (1992) 591-600.
[2]
Ph. Souplet, Blow up in nonlocal reaction-diffusion equations, SIAM J. Math. Anal. 29(1998), 1301-1334.
[3]
Ph. Souplet, Uniform blow-up profiles and boundary behavior for diffusion equations with nonlocal nonlinear source, J. Differential Equations 153 (1999) 374-406.
[4]
Y. P. Chen, Q. Liu, C. H. Xie, Blow-up for degenerate parabolic equations with nonlocal source, Proc. Am. Math. Soc. 132 (1) (2004) 135-145.
[5]
W. B. Deng, Z. W. Duan, C. H. Xie, The blow-up rate for a degenerate parabolic equation with a nonlocal source, J. Math. Anal. Appl. 264 (2001) 577-597.
[6]
Zhoujin Cui, Zuodong Yang, Roles of weight functions to a nonlinear porous medium equation with nonlocal source and nonlocal boundary condition, J. Math. Anal. Appl. 342 (2008) 559-570.
[7]
Zhoujin Cui, Zuodong Yang, Uniform blow-up rates and asymptotic estimates of solutions for diffusion system with nonlocal sources, Differential Equations and Nonlinear Methanics, 2007, Article ID 87696: 1-16.
[8]
M. X. Wang, Y. M. Wang, Properties of positive solutions for nonlocal reaction-diffusion problems, Math. Methods Appl. Sci. 19 (1996) 1141-1156.
[9]
M. S. Floater, Blow-up at the boundary for degenerate semilinear parabolic equations, Arch. Rat. Mech. Anal. 114 (1) (1991) 57-77.
[10]
C. Y. Chan, H. T. Liu, Global existence of solutions for degenerate semilinear parabolic problems, Nonlinear Anal. 34 (4) (1998) 617-628.
[11]
H. Ockendon, Channel flow with temperature-dependent viscosity and internal viscous dissipation, J. Fluid Mech. 93 (1979) 737-746.
[12]
A. Friedman and B. McLeod, Blow-up of positive solutions of semilinear heat equations, Indiana University Mathematics Journal 34 (1985), 425-447.
[13]
C. Budd, V. A. Galaktionov, and J. Chen, Focusing blow-up for quasilinear parabolic equations, Proceedings of the Royal Society of Edinburgh. Section A 128 (1998), 965-992.
[14]
A. A. Samarskii, V. A. Galaktionov, S. P. Kurdyumov, and A. P. Mikhailoi, Blow-up in Qusilinear Parabolic Equations, Nauka, Moscow, 1987.
[15]
Q. L. Liu, Y. P. Chen, C. H. Xie, Blow-up for a degenerate parabolic equation with a nonlocal source, J. Math. Anal. Appl. 285 (2003) 487-505.
[16]
Congming Peng, Yang Zuodong, Blow-up for a degenerate parabolic equation with a nonlocal source, Applied Mathematics and Computation 201 (2008) 250-259.


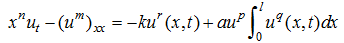 in
in 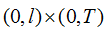 where
where